What is Quantum finance?
Did you know that the same quantum principles powering supercomputers now shape our understanding and prediction of financial markets?
Quantum finance brings a fresh perspective to traditional financial methods. The field applies quantum mechanics principles to tackle complex financial challenges. This blend of quantum physics, mathematics, and financial theory leads to more accurate market predictions and risk assessments.

This piece breaks down quantum finance and shows how it works. You’ll discover its core principles and see its real-world applications in trading and risk management. The future of financial analysis lies in this innovative field. We’ll help you learn these concepts in simple terms, whether you’re a finance professional or just curious about this emerging area.
Understanding Quantum Finance Basics
The fundamental building blocks of quantum finance merge quantum physics principles with financial mathematics to create powerful new tools for market analysis.
Definition and Core Principles
Quantum finance represents an interdisciplinary research field that applies theories and methods from quantum physics to solve complex financial problems. The field operates on several key principles:
- Quantum Superposition: This concept allows analysis of multiple investment scenarios simultaneously and provides a more complete view of potential market outcomes
- Entanglement Theory: This helps understand how different financial instruments connect and influence each other
- Wave Function Applications: These models help track market behavior and price movements with greater precision
Development from Traditional Finance
Quantum finance emerged as a distinct discipline in the 1970s after groundbreaking work by Fischer Black, Myron Scholes, and Robert Merton on option pricing theory. The field expanded beyond traditional mathematical finance that relied on classical computational methods.

What is Microfinance
This development changed our approach to financial modeling. Traditional quantitative finance focused on customer-driven derivatives and structured products. Quantum finance brought more sophisticated tools to analyze market behavior and assess risks.
Key Mathematical Concepts
Several sophisticated tools and theories form the mathematical foundation of quantum finance:
- Path Integrals: These tools, based on Richard Feynman’s work, help analyze exotic options and compare results with traditional Black-Scholes-Merton equations
- Quantum Field Theory: This framework provides mathematical tools to model complex financial systems and market behaviors
- Schrödinger Equation: Research found that the Black-Scholes-Merton equation represents a special case of the Schrödinger equation, especially when markets show efficiency
These mathematical concepts prove powerful because they handle scenarios where traditional market efficiency assumptions fail. To name just one example, Haven’s research shows that quantum option pricing models can deliver more accurate results than classical approaches, especially in inefficient markets.

The mathematical frameworks now solve complex financial problems that traditional methods couldn’t handle. This includes improved portfolio optimization, precise risk assessment models, and sophisticated trading algorithms that process multiple market scenarios at once.
Quantum Mechanics in Finance
Quantum finance applies fundamental principles of quantum mechanics to understand complex market behaviors. This fresh perspective lets us analyze financial markets with unprecedented precision.
Wave Functions in Financial Markets
Scientists found that there was a way to use wave functions from quantum physics to model financial market behavior. Just as quantum particles exist in multiple states simultaneously, market prices and trends can be represented as probability waves. These wave functions help us:
- Model price fluctuations more accurately
- Predict market trends with greater precision
- Analyze multiple market scenarios simultaneously
- Calculate option prices with improved accuracy
Heisenberg Uncertainty Principle Applications
The sort of thing I love is the parallel between Heisenberg’s uncertainty principle and financial markets. Just as we cannot measure a particle’s position and momentum with perfect accuracy at once, similar limitations exist in finance. Our measurement of one market variable affects the certainty of measuring related variables.

To cite an instance, see what happens when analysts try to determine both price and momentum in markets with high precision. They face fundamental limitations like those described by Heisenberg’s principle. This insight has led to more realistic risk assessment models that factor in natural market uncertainties.
Quantum Probability Theory
Quantum finance uses probability theory differently from classical finance. Traditional probability models assume market efficiency, but quantum probability theory accounts for market inefficiencies and anomalies. This approach proves valuable in:
- Market State Analysis: Models can now represent multiple market states simultaneously and provide a full picture of potential outcomes.
- Risk Assessment: Quantum probability models help identify hidden risks by factoring in quantum interference effects.
- Trading Decisions: Quantum probability distributions lead to better-informed trading decisions, especially in volatile markets.
Research shows that the Black-Scholes-Merton equation, central to traditional finance, represents a special case of the Schrödinger equation. This revelation creates new possibilities for financial modeling and risk assessment.
These quantum mechanical principles have revolutionized financial analysis tools. They work especially well when traditional models struggle during high market volatility or with complex financial instruments.
Quantum Computing Applications
Quantum computing brings groundbreaking changes to finance. These changes reshape complex financial calculations and decision-making processes in ways we never imagined possible.
Portfolio Optimization
Quantum computing has made remarkable strides in portfolio optimization, especially when you have the Harrow-Hassidim-Lloyd (HHL) algorithm. Research shows this algorithm works effectively to solve portfolio optimization problems by converting them into quantum-compatible linear systems. Tests on Quantinuum System Model H-series trapped-ion quantum computers have yielded high-quality solutions that match noiseless simulations closely.
Read More:
Recent implementations show quantum portfolio optimization handles larger, more complex portfolios effectively. Our quantum algorithm yielded impressive results with a portfolio of 78 stocks, achieving a 0.07% return under a 1% risk threshold. This shows how quantum computing processes more variables at once compared to traditional methods.
Risk Assessment Models
Quantum computing has transformed risk assessment through advanced simulation techniques. Quantum Monte Carlo methods show remarkable efficiency when simulating complex financial systems and calculating vital risk measures like Value-at-Risk (VaR) and Conditional Value-at-Risk (CVaR).
Quantum risk assessment excels because it can:
- Process big amounts of market data immediately
- Model multiple market scenarios at once
- Identify potential risks and tail risks more accurately
Trading Algorithms
Our trading systems now blend quantum algorithms to improve decision-making capabilities. The Quantum Approximate Optimization Algorithm (QAOA) works particularly well to analyze extensive datasets and identify market patterns. We see notable improvements in:
- Pattern Recognition: Quantum algorithms detect market trends and anomalies with unprecedented precision
- Speed Optimization: Market data processing happens in real time
- Enhanced Predictive Models: Systems develop sophisticated market trend forecasts
Quantum computing shines when handling tasks that would take traditional computers years. Our quantum-enhanced trading systems analyze multiple market scenarios simultaneously, which leads to better decisions in complex market conditions.
Quantum Price Theory
Our research into quantum price theory shows fascinating connections between quantum mechanics and financial markets. The data reveals that stock prices display both wave and particle properties, much like quantum systems.
Price Level Modeling
A groundbreaking approach to modeling price levels uses quantum wave functions. The square modulus of the wave function represents price distribution in our model. Each point maps to potential stock prices at specific moments. This innovative method lets us:
- Track price fluctuations with exceptional precision
- Model multiple price scenarios at once
- Calculate probability distributions for future price movements
- Measure market uncertainty immediately
Market State Functions
Market states mirror quantum systems remarkably. A stock’s state before trading exists as a wave packet – a superposition of various possible prices. External factors like investor decisions to buy or sell behave like physical measurements in quantum mechanics.
Economic quantities appear as Hermitian operators in Hilbert space within our quantum finance framework. This mathematical structure reveals how:
- Market capitalization drives price movement speed
- Trading volumes shape state transitions
- External information shapes price progress
- Market momentum links to price uncertainty
Quantum Interference Effects
Quantum interference plays a vital role in market behavior. Market movement analysis must account for all possible paths of price progress. The Hamiltonian in our model factors in:
- Economic environment influences
- Market information flow
- Investor psychology
- External field effects from information
External information creates periodic effects on market states, similar to electromagnetic fields affecting charged particles. Information fields can trigger distribution imbalances at specific time points, which lead to measurable market effects.
Quantum interference analysis provides deep insights into market force interactions. The average rate of return calculations now use quantum mechanical principles, expressed through an integral over all possible price states. This advancement helps predict market behavior and explains the intricate relationships between various financial factors.
Mathematical Tools and Methods
Our exploration of quantum finance’s mathematical foundations reveals powerful tools that connect quantum physics and financial analysis. These sophisticated methods help analysts understand market behavior at a fundamental level.
Quantum Field Theory Basics
Quantum field theory (QFT) provides a framework that models financial markets effectively. QFT combines classical field theory, quantum mechanics, and special relativity to explain complex market behaviors. Our research uses QFT to:
- Model market fluctuations
- Analyze particle-like behavior of financial instruments
- Study field interactions in market dynamics
- Apply quantum mechanical principles in financial modeling
Schrödinger Equation in Finance
The Schrödinger equation solves complex financial problems effectively. This equation describes particle behavior in force fields and changes in physical quantities over time. Financial applications allow us to:
- Calculate wave functions that control market movements
- Determine quantum numbers for financial modeling
- Analyze orbital shapes where market patterns emerge
- Study time-dependent market behaviors
The equation works well with time-dependent and time-independent scenarios in financial markets. Research shows that the Schrödinger equation explains multiple orbitals and fine spectra in various market conditions effectively.
Statistical Methods
Quantum finance applications make use of advanced statistical techniques that blend traditional and quantum approaches. The statistical toolkit has several sophisticated methods to analyze financial data:
Time Series Analysis
- ARIMA modeling for financial forecasting
- Exponential smoothing for short-term predictions
- GARCH models for volatility analysis
Risk Management Tools
- Value at Risk (VaR) calculations
- Monte Carlo simulations
- Conditional VaR for tail risk assessment
Portfolio Theory Applications
- Mean-variance optimization
- Capital Asset Pricing Model implementations
- Sharpe ratio calculations
These statistical methods combined with quantum principles yield more accurate results than classical approaches alone. Regression analysis and hypothesis testing remain fundamental tools, yet their application in quantum finance offers better precision and predictive power.
The integration of quantum field theory, Schrödinger’s equation, and advanced statistical methods creates more accurate models for price prediction and risk assessment.
Risk Management in Quantum Finance
Quantum principles combined with traditional risk assessment methods bring a fresh dimension to financial risk management. Our quantum finance research shows new ways to understand and control market uncertainties.
Quantum Risk Measures
We developed sophisticated risk measures that blend quantum principles with traditional financial metrics. The Sharpe ratio is a vital tool in our quantum risk assessment framework. It helps us assess the returns generated against risks taken. Our analysis shows that:
- Portfolio performance assessment using quantum-enhanced metrics
- Risk calculation through standard deviation measurements
- Return optimization relative to risk-free rates
- Negative Sharpe ratio identification for underperforming investments
Quantum computing substantially improves our risk assessment models by providing more accurate market behavior predictions. Our quantum algorithms now improve Monte Carlo simulations. This leads to better estimation of risk factors and more resilient stress testing.
Uncertainty Principles
Our quantum finance work found striking parallels between Heisenberg’s Uncertainty Principle and financial risk assessment. We cannot measure a particle’s position and momentum with perfect precision. The same limitations apply to financial risk calculations.
Risk management in quantum finance handles multiple parameters at once, which makes accurate measurement challenging. Our research shows a Risk Management Framework needs careful attention to:
- Risk appetite determination
- Stakeholder viewpoint lineup
- Syndication elements
- Taxonomy development
Hedging Strategies
Our advanced hedging strategies use quantum principles to protect investment portfolios. The hedging approach in quantum finance takes opposite positions to reduce potential losses. Our research identified several effective hedging techniques.
Options and derivatives play a key role in hedging strategies. Put options protect against stock price declines by offsetting potential losses in primary holdings. This strategy works well for:
- Stabilizing cash flow and income
- Improving long-term planning capabilities
- Protecting against adverse price movements
- Distributing and reducing overall risk
Quantum-enhanced hedging strategies help balance risk and return better. Good hedging can secure profits by protecting investments from sudden market downturns. The quantum approach analyzes multiple market scenarios simultaneously and implements sophisticated hedging strategies.
These strategies show that quantum computing improves our ability to process complex market data. This capability leads to more resilient risk management strategies and better loss reduction. Our research shows quantum-based risk assessment models handle modern financial market complexity more efficiently than traditional approaches.
Quantum Trading Systems
Quantum computing and algorithmic trading have revolutionized how trades happen in modern markets. Our analysis reveals that algorithmic trading makes up about 92% of Forex market trades. This shows how automated systems now dominate today’s financial world.
Algorithmic Trading Platforms
Trading platforms have grown by a lot as they merge quantum capabilities. Our trading systems now mix classical algorithms with quantum computing to boost speed and accuracy. These platforms give traders several advantages:
- Up-to-the-minute data analysis and pattern recognition
- Boosted portfolio optimization capabilities
- Better risk assessment models
- Automated trade execution with quantum precision
- Advanced backtesting capabilities
Quantum algorithms, especially the Quantum Approximate Optimization Algorithm (QAOA), have boosted our knowing how to analyze so big amounts of market data and optimize trading strategies.
Quantum-Based Decision Making
Our quantum-based decision systems now include advanced pattern recognition that processes market data at never-before-seen speeds. Quantum computing streamlines complex calculations and cuts computation time from days to seconds.
Our quantum decision-making framework follows these steps
- Data Collection: Getting market information as it happens
- Quantum Processing: Looking at multiple market scenarios at once
- Pattern Recognition: Finding trading opportunities with quantum algorithms
- Risk Assessment: Checking what it all means using quantum probability models
- Trade Execution: Making trades through automated systems
We use क्वांटम कंप्यूटर architecture to process multiple states at once through क्यूबिट्स. This quantum edge lets us analyze complex market scenarios faster than traditional computing systems.
System Architecture
Our quantum trading system mixes both classical and quantum parts. The system handles quantum computing challenges while keeping trading platforms reliable.
The design supports high-frequency trading (HFT) that needs microsecond-level speeds. Electronic communication networks (ECNs) make trading outside traditional exchanges easier and boost our market access.
Quantum computers need specific conditions to work their best. Our reliable error-correction protocols and backup systems address these needs.
Advanced monitoring tools track how well everything works and what’s happening in the market right now. Strong security features protect against cyber threats and keep trading operations safe. Quantum-enhanced systems now process market data and execute trades faster and more accurately than ever.
AI and machine learning algorithms power our quantum trading systems. This combination helps scan markets better and find trades that match specific risk priorities. The systems learn from every trade and adapt to market changes to work better over time.
Future Prospects and Challenges
The future of quantum finance shows incredible potential despite major hurdles. A closer look at this emerging field reveals opportunities and challenges that will reshape its progress.
Technological Limitations
Quantum computing hardware presents several roadblocks that slow our progress. The biggest problem lies in high error rates in quantum systems, which need complex error correction techniques. Quantum decoherence remains a major obstacle because qubits react very easily to environmental factors like radiation, light, sound, vibrations, and heat.
These key technical constraints stand out:
- Qubit stability maintenance in different conditions
- Resource-heavy nature of quantum hardware
- Scalability problems in larger systems
- Need for reliable error correction methods
Quantum computers need specialized conditions, including ultra-low temperatures and controlled environments, which lead to high operational costs.
Research Opportunities
Research opportunities are booming, especially in India. The government has pledged ₹8,000 crore over five years for quantum technology research. This shows strong national support for the field’s advancement. IIT Madras received ₹21 crore specifically for quantum computing research.
Research priorities target:
- Quantum optimization techniques
- Advanced financial modeling systems
- Error correction methodologies
- Quantum communication protocols
- Machine learning integration
About 75% of industry leaders see quantum as a game-changing technology. Studies show 74.5% of experts stress the need for more investment in quantum innovation.
Industry Adoption
Financial services lead the charge in quantum computing adoption. Data shows 14 out of 70 early adopters come from this sector. Major institutions like AXA, HSBC, Goldman Sachs, JPMorgan Chase, Mastercard, and Wells Fargo already test quantum applications.
The adoption landscape shows these patterns:
- 32.6% of industry leaders expect practical quantum applications within 3-5 years
- 27.7% think widespread deployment will take over five years
- 71% see high R&D costs as a major obstacle
- 62% point to skilled talent shortage as a barrier
Quantum computing in finance focuses on fraud detection and portfolio optimization. Research shows 63% of experts believe it will improve financial systems’ efficiency and security.
The job market needs specialized talent. IBM offers annual salaries from INR 1,44,000 to INR 5,57,000 for quantum computing roles. Most positions need advanced degrees, typically a PhD in physics, computation, astronomy, or information science.
These sectors will benefit most from quantum adoption:
- AI and machine learning (79.4% of experts agree)
- Cybersecurity and cryptography (68.1%)
- Healthcare and drug discovery (61%)
Collaborative initiatives across sectors show promise. About 45% of leaders stress the importance of partnerships between governments, private sector, and international organizations. This teamwork helps overcome financial and talent-related barriers to quantum growth.
Quantum finance will develop in phases. Hybrid models combining classical and quantum computing will come first. As technology matures, fully quantum solutions will emerge, especially in risk assessment and predictive analytics.
Quantum finance combines quantum physics with financial mathematics and changes how we analyze and predict market behavior. Quantum principles like superposition and entanglement now create powerful tools that revolutionize financial analysis.
Our research shows these innovative achievements:
- Quantum computing applications solve complex portfolio optimization problems in seconds
- Advanced risk assessment models use quantum probability theory to predict more accurately
- Mathematical tools from quantum mechanics help us learn about market behavior
- Trading systems powered by quantum algorithms process big amounts of data at once
Hardware limitations and error correction remain key challenges. However, big investments and growing industry acceptance show strong momentum. Many financial institutions already use quantum applications to detect fraud and optimize portfolios. Research initiatives, especially in India, expand possibilities with strong funding support.
Quantum finance will progress through hybrid models before moving to fully quantum solutions. This change will bring sophisticated financial analysis tools, better risk management, and new computational power to predict markets.
The quantum revolution has started to revolutionize finance. It will change how we understand and work with financial markets. Scientists and financial experts collaborate globally to advance this field that connects the microscopic quantum world with global financial systems.
FAQs
क्वांटम फाइनेंस क्या है और यह पारंपरिक वित्त से कैसे भिन्न है?
क्वांटम फाइनेंस क्वांटम भौतिकी के सिद्धांतों को वित्तीय समस्याओं पर लागू करने वाला एक अंतःविषय क्षेत्र है। यह जटिल बाजार व्यवहारों को समझने और भविष्यवाणी करने के लिए क्वांटम सुपरपोजिशन और एंटैंगलमेंट जैसी अवधारणाओं का उपयोग करता है, जो पारंपरिक वित्तीय मॉडलों की तुलना में अधिक सटीक परिणाम प्रदान करता है।
क्वांटम फाइनेंस में जोखिम प्रबंधन कैसे किया जाता है?
क्वांटम फाइनेंस में जोखिम प्रबंधन पारंपरिक उपायों और क्वांटम सिद्धांतों का संयोजन है। यह शार्प अनुपात जैसे उन्नत मेट्रिक्स का उपयोग करता है और क्वांटम-वर्धित मोंटे कार्लो सिमुलेशन का उपयोग करके अधिक सटीक जोखिम आकलन प्रदान करता है। इसके अलावा, यह हेजिंग रणनीतियों को लागू करने के लिए क्वांटम कंप्यूटिंग का लाभ उठाता है जो एक साथ कई बाजार परिदृश्यों का विश्लेषण कर सकते हैं।
क्वांटम ट्रेडिंग सिस्टम कैसे काम करते हैं?
क्वांटम ट्रेडिंग सिस्टम क्लासिकल और क्वांटम कंप्यूटिंग को जोड़ते हैं। वे क्वांटम एल्गोरिदम का उपयोग करके वास्तविक समय में बाजार डेटा का विश्लेषण करते हैं, पैटर्न की पहचान करते हैं, और मिलीसेकंड में निर्णय लेते हैं। ये सिस्टम कई बाजार परिदृश्यों का एक साथ विश्लेषण कर सकते हैं, जो उन्हें पारंपरिक ट्रेडिंग प्लेटफॉर्म की तुलना में अधिक कुशल बनाता है।
क्वांटम फाइनेंस के लिए किन गणितीय उपकरणों का उपयोग किया जाता है?
क्वांटम फाइनेंस कई उन्नत गणितीय उपकरणों का उपयोग करता है। इनमें क्वांटम फील्ड थ्योरी, श्रोडिंगर समीकरण, और उन्नत सांख्यिकीय विधियां शामिल हैं। ये उपकरण जटिल बाजार व्यवहार को मॉडल करने, मूल्य उतार-चढ़ाव की भविष्यवाणी करने और जोखिम का अधिक सटीक आकलन करने में मदद करते हैं।
क्वांटम फाइनेंस के भविष्य की संभावनाएं और चुनौतियां क्या हैं?
क्वांटम फाइनेंस का भविष्य आशाजनक है, लेकिन चुनौतियों से भरा है। प्रमुख संभावनाओं में बेहतर पोर्टफोलियो अनुकूलन, सटीक जोखिम आकलन और उन्नत व्यापारिक एल्गोरिदम शामिल हैं। हालांकि, प्रौद्योगिकी सीमाएं जैसे क्वांटम हार्डवेयर में उच्च त्रुटि दरें और डीकोहेरेंस प्रमुख चुनौतियां हैं। इसके अलावा, उद्योग अपनाने और कुशल प्रतिभा की कमी भी महत्वपूर्ण बाधाएं हैं जिन्हें दूर करने की आवश्यकता है।
क्वांटम वित्त का क्या अर्थ है?
इसका संक्षिप्त परिभाषा यह है: क्वांटम अर्थशास्त्र और वित्त प्रोजेक्टिव ज्यामिति पर आधारित संभाव्यता का उपयोग है, जिसे क्वांटम संभाव्यता भी कहा जाता है, जो अर्थशास्त्र और वित्त में मॉडलिंग के लिए लागू होता है। यह क्वांटम संज्ञान, क्वांटम खेल सिद्धांत, क्वांटम कंप्यूटिंग और क्वांटम भौतिकी जैसे संबंधित क्षेत्रों से प्रेरित है।
वित्त में क्वांटम सिद्धांत क्या है?
क्वांटम सिद्धांत का उपयोग द्वितीयक वित्तीय बाजारों के मॉडलिंग के लिए किया जाता है। यादृच्छिक वर्णनों के विपरीत, यह औपचारिकता व्यापार के महत्व को सुरक्षा के मूल्य निर्धारण में उजागर करती है। निवेशकों द्वारा सुरक्षा और नकद रखने के सभी संभावित रूपों को बाजार स्थितियों के हिल्बर्ट स्पेस का आधार माना जाता है।
क्वांटम फाइनेंशियल्स क्या है?
क्वांटम फाइनेंशियल्स एक क्लाउड-आधारित वित्तीय समाधान है जिसमें स्वचालन और कॉन्फ़िगर करने योग्य कार्यप्रवाह शामिल हैं, जो वित्तीय डेटा का उपयोग करके व्यावसायिक निर्णय लेने की क्षमता प्रदान करता है।
क्वांटम फाइनेंशियल का मालिक कौन है?
Bill Mackay established Quantum Financial in 1994 after over 30 years experience in financial services in Australia and the USA.

Family ID UP: Login, Registration, Status

Bhumi ko Samagra ID se link kre
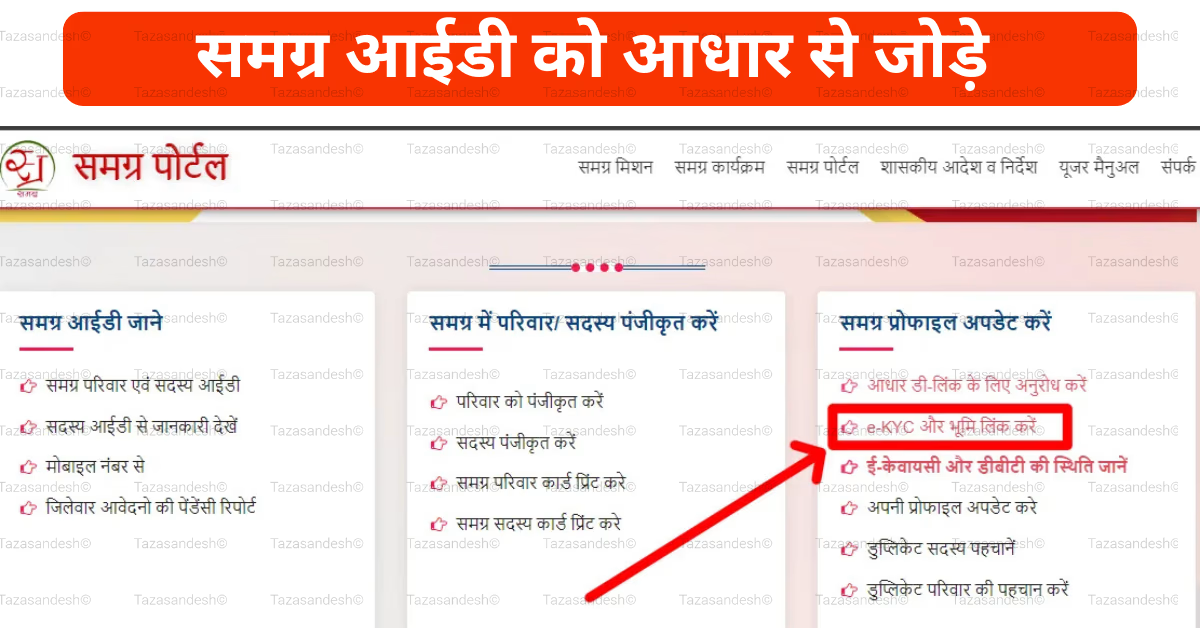
Samagra ID eKYC mp
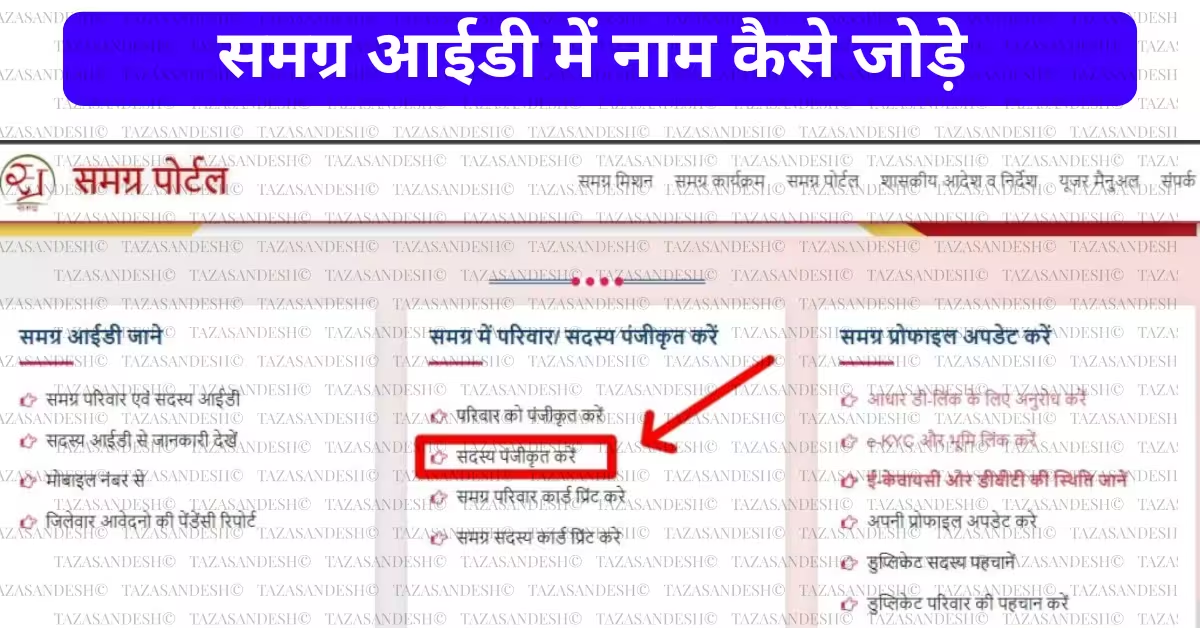
Samagra ID member registration

Samagra Portal Sadasya ID

Samagra ID Search by name

Samagra ID Portal MP: Registration, e-kyc, NPCI Status

APAAR ID Card Apply Online



